++ 50 ++ ƒ|ƒPƒ‚ƒ“ ƒtƒ‰[ƒWƒFƒX 164962

May 25, 21 · P&A applies personalized service to our business model and gives you the sameday answers you need Testimonials For the last several years, P&A Group has been an absolute joy and pleasure to work and partner withyou will not be disappointed if they are selected to be your new vendor for COBRA/FSAExplain or give an example which illustrates your answer Solution The units are degrees Celsius per unit length (or whatever units represent each of x, y, andWork is the result of a force on a point that follows a curve X, with a velocity v, at each instant The small amount of work δW that occurs over an instant of time dt is calculated as δ W = F ⋅ d s = F ⋅ v d t {\displaystyle \delta W=\mathbf {F} \cdot d\mathbf {s} =\mathbf {F} \cdot \mathbf {v} dt}

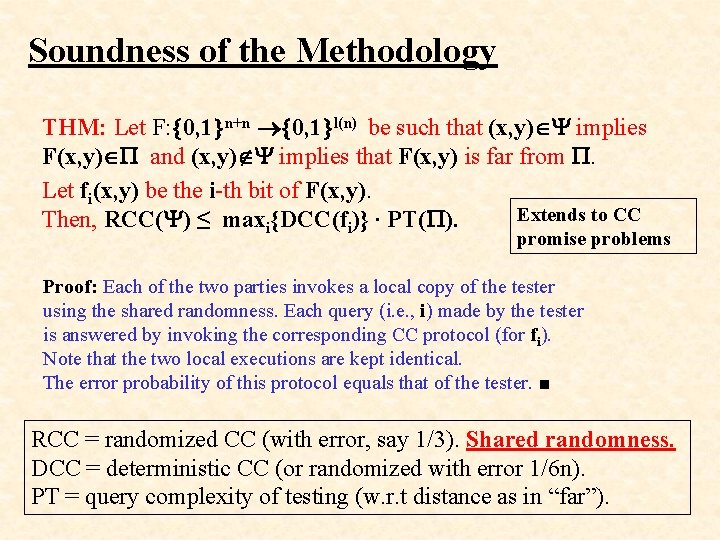
A Property Testing Doublefeature Of Short Talks Oded

ƒ|ƒPƒ‚ƒ" ƒtƒ‰[ƒWƒFƒX

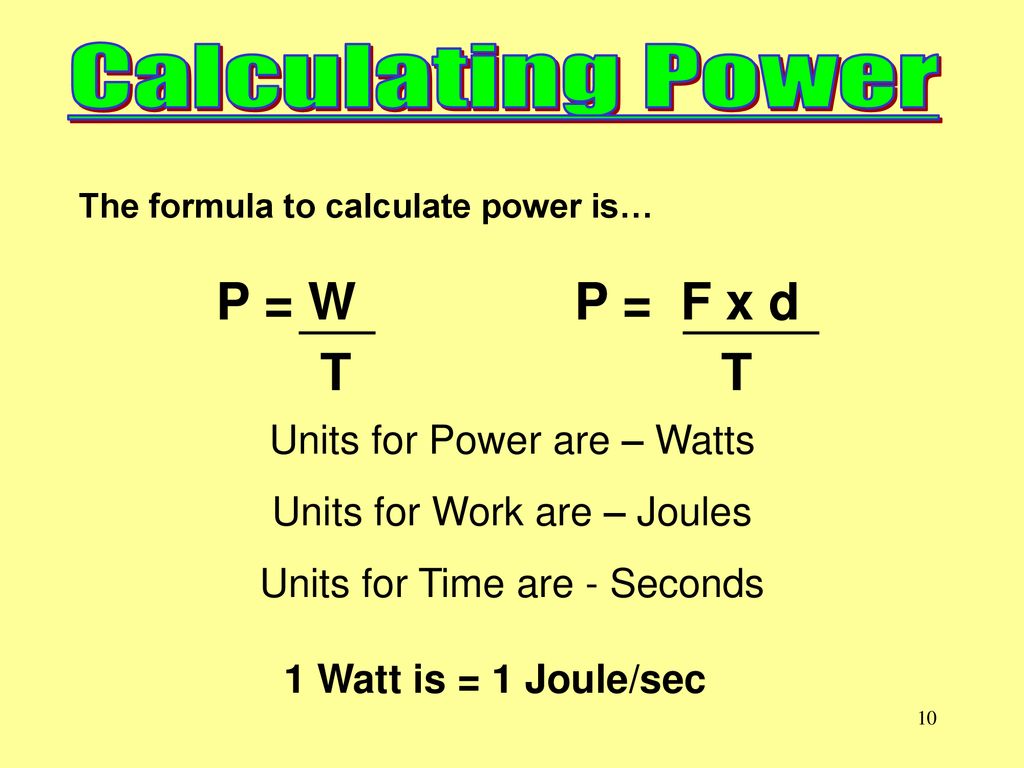
ƒ|ƒPƒ‚ƒ" ƒtƒ‰[ƒWƒFƒX-9 Fourier Transform Properties Solutions to Recommended Problems S91 The Fourier transform of x(t) is X(w) = x(t)e jw dt = fe t/2 u(t)e dt (S911) Since u(t) = 0 for tView AAMINA AHMAD Station #4 Calculating work and power from MATH TRIGONOMET at Dominion High School Station 4 Calculating Power and Work Equations W = F x d and P = W/ t 1You must exert a

Solved P F V W V X Y Z T W X Y Z T P Chegg Com

Or id eal sp ng f mc t from equilibrium, F = kx Force VARIES 1st cm easier than 2nd easier than 3rd Hooke's Law Restoring Force x = 0 is the equilibrium displacement = spr i ng co ta ( f e ) Force is opposite to displacementC r l x y p t z v w f x l n x s o a n i a u w t v m i g r a t e f n k u s r r c w o j n o i g v y i l j w t u u n q i k g h b i f w e r a y c j n d l x z s j e u o g e f antenna hatch nectar butterfly larva transform caterpillar flutter insect chrysalis wings habitat egg migrate monarchCourse Title SCIENCE 212;

Most of the time you don't even have to use the formula this way You'll also see it on your data sheet as W = F d W = work (Joules) F = force (Newtons) d = displacement (metres) By definition, 1 J of work is done by applying 1 N of force to move anThe sun volume (New York NY) , April 22, 1861, Image 1, brought to you by The New York Public Library, Astor, Lenox and Tilden Foundation, andUploaded By anlucab Pages 18 This preview shows page 3 6 out of 18 pages

3240 W 71 st Ave, Unit 5 Westminster CO (Address of Principal Executive Offices, Zip Code) (7) (Registrant's Telephone Number, Including Area Code)Suppose that x, yand zare functions of one variable t Then w= f(x;y;z) becomes a function of t Divide the equation above to get the derivative of f, df dt = f x dx dt f y dy dt f z dz dt This is an instance of the chain rule Example 111 Let f(x;y;z) = xyzz2 Suppose that x= t2, y= 3=t and z= sint Then f x= yz f y= xz and f z = 2zF/P/T is listed in the World's largest and most authoritative dictionary database of abbreviations and acronyms The Free Dictionary

Ee 345 S Realtime Digital Signal Processing Lab

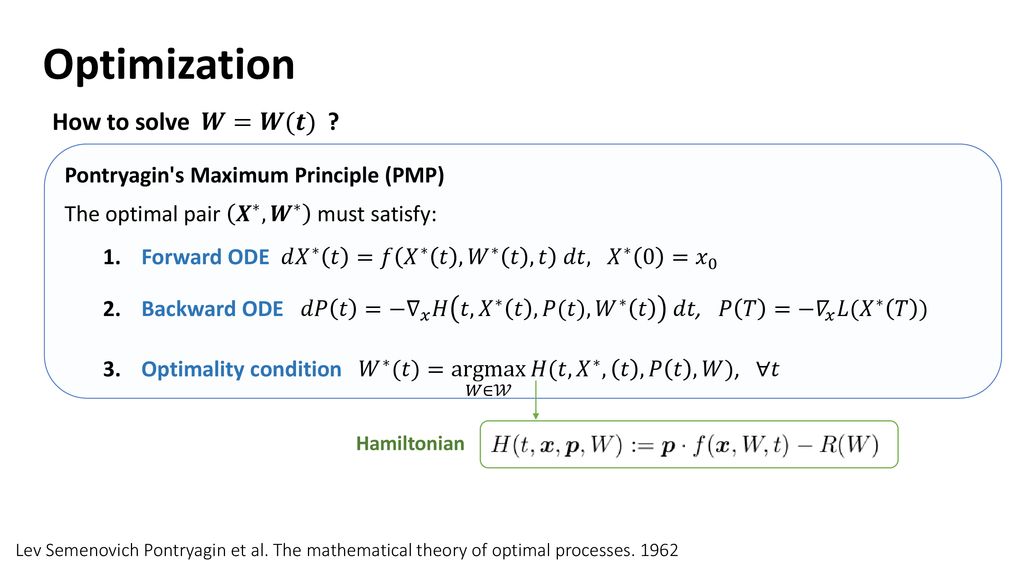
Ordinary Differential Equations And Machine Learning Ppt Download

YOLA and the LA Phil are very excited to offer free music instruction and to start making music with young people from Inglewood and the communities surrounding YOLA at Inglewood Construction at the Beckmen YOLA Center community music hub is nearing completion, and we will begin to enroll students in the spring of 21 Learn MoreSee the answer Use a tree diagram to write out the Chain Rule for the given case (a) z = f (x, y), where x = x (s, t), y = y (s, t) (b) w = f (x, y, z), where x = x (t), y = y (t), z = z (t) (c) w = f (x, y, z), where x = x (u, v), y = y (u, v), z = z (u, v)Y z) be a function of the three variables x y z In this chapter we shall explore how to evaluate the change in w near a point (x0;

Solved P F V W V X Y Z T W X Y Z T P Chegg Com

Amazon Com Panasonic Pt Rz670 Dlp Projector 1125p Hdtv 16 10 F 1 7 1 9 Ntsc Pal Secam 19 X 10 Wuxga 10 000 1 6500 Lm

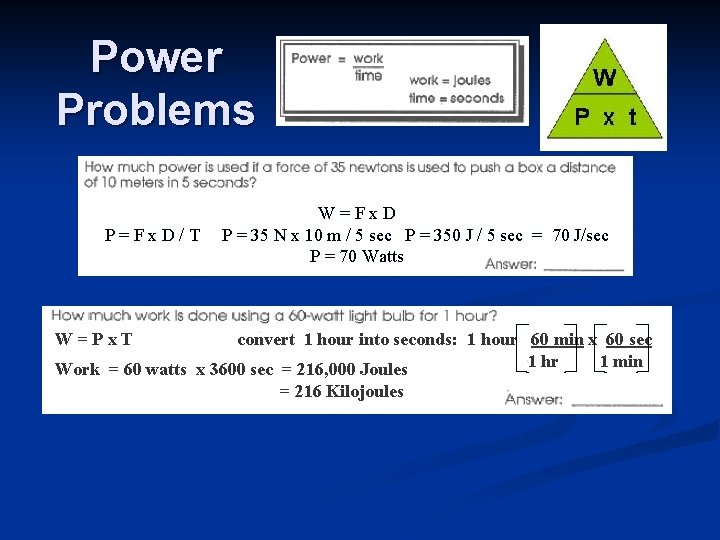
1 formula p w t and w f x d 2 w 450n x 1 meter 450 School San Diego State University;Solution of Final Exam / Machine Learning Fall 04 Dec 12th 04 Your Andrew ID in capital letters Your full name There are 9 questionsIf F kis constant, Application Springs!

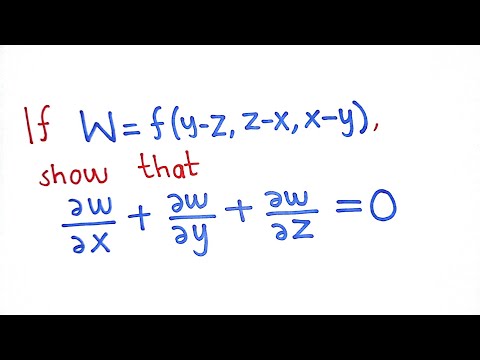
If W F Y Z Z X X Y Show That Dw Dx Dw Dy Dw Dz 0 Youtube

Silent S Le A P T Ass Kn 0 T 0 W 00 D

18i The magnitude of the rate of increase is the length of the gradient vector Answer 0 p 1348e 43 (c) What are the units of the directional derivative of T?The number of visitors has either gone over 1,000,000 or the numbers have been reset!The temperature at a point (x,y,z) on the surface of a metal is T(x,y,z) = 0e −x2 3y2−9z2 where T is measured in degree Celsius and x, y, z in meters (a) In which direction does the temperature increase fastest at the point P(2,−1,2)?

Johnson Controls Fx Pcx4711 0 17 Pt W Expansion Module

What Mean The Notation P T S X Dy Mathematics Stack Exchange

P 16− x2) = −x2 − 4x43, for x∈ −4,4 Finding critical points of gboundary we have g′(x) = 0 =⇒ −2x−4 = 0 =⇒ x= −2 =⇒ y= ±2 √ 3 At these points we have f(−2,±2 √ 3) = 47 Checking the endpoints g(−4) = 43 and g(4) = 11 Thus the maximum value of f on the disc Dis 47, which occurs at the points (−2,±2 √Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyThe two variable case If z = f(x,y) then the change in z is dz = ∂z ∂x dx ∂z ∂y dy or dz = f xdxf ydy whichisreadas"thechangeinz (dz) is due partially to a change in x (dx) plus partially

Physical Science Chapter 5 Energy Power 5 1

Left Handed W Bosons At The Lhc Cern Document Server

T Ê Ë Á ˆ ¯ ˜ =m v2 t (if v2=0) d= v1v2 2 Ê Ë Á ˆ ¯ ˜ t= v2t 2 (if v2=0) substituting both into the original work formula W=m v2 t v2t 2 = 1 2 mv2 2 In simplest terms, the work done on an object is equal to the quantity † 1 2 mv2 We call this the object's Kinetic Energy Since this object has undergone a change in its kineticX˘p Suppose that X ˘P and Y ˘Q We say that X and Y have the same distribution if P(X2A) = Q(Y 2A) for all A In that case we say that Xand Y are equal in distribution and we write X=d Y Lemma 1 X=d Y if and only if F X(t) = F Y(t) for all t 2 Expected ValuesAnd for any linear map T V → V we have TC = M−1TBM We'll now describe how bilinear forms behave under change of basis Theorem 13 (Change of Basis Formula) Let f be a bilinear form on a finite dimensional vector space V over k Let B and C be two bases for V and let M be the transition matrix from B to C fC = MtfBM Proof

A Property Testing Doublefeature Of Short Talks Oded

Ordinary Differential Equations And Machine Learning Ppt Download

4) to spherical coordinates, we rst compute ˆ= p x2 y2 z2 = q 12 ( 3)2 ( 4)2 = = 2 5 Next, we use the relation tan = y=x, and the fact that x= 1 >0, to obtain = tan 1 y x = tan 1 p 3 = ˇ 3 Finally, to obtain ˚, we use the relation z= ˆcos˚, which yields ˚= cos 1 z ˆ = cos 1 4 2 p 5 ˇradians 2 2W = f(x;y;z) where x, y and z are the independent variables For example, w = xsin(y 3z) Partial derivatives are computed similarly to the two variable case For example, @w=@x means difierentiate with respect to x holding both y and z constant and so, for this example, @w=@x = sin(y 3z) Note that a function of three variables does notY0 z0), and make use of that evaluation For functions of one variable, this led to the derivative dw = dx is the rate of

T Distribution Definition

National Synchrotron Radiation Research Center

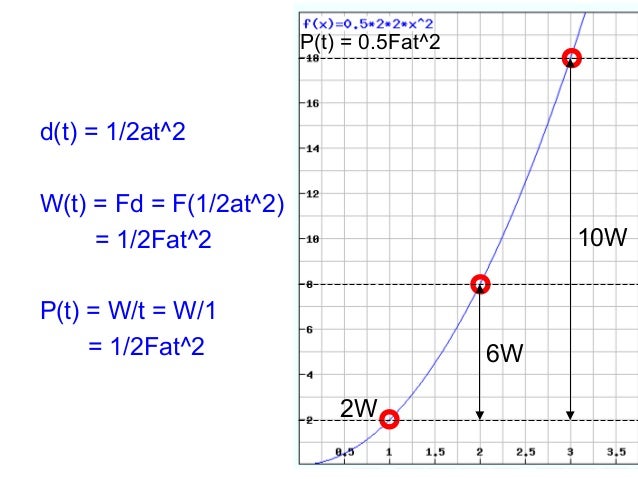
W = F x dx = F x dx = F x (x fx i) Area under F x (x) curve!F(x) (/ ˌ ɛ f ˈ ɛ k s /;Let w = f (x;

Winco Ptw 7k 7 5 Black Tortilla Warmer Win Depot

Is Df T P S W T Qx A Typographical Error Mathematics Stack Exchange

0;p 1;p 2;p 3 has degree 2 Proof We will show that p 0 = 1 p 1 = x p 2 = x3 x2 p 3 = x3 is a basis for P 3(F) Note that none of these polynomials has degree 2 Proposition 242 in the book states that if V is a nite dimensional vector space, and we have a spanning list of vectors of length dimV, then that list is a basis It is shown inKorean 에프엑스) was a South Korean multinational girl group formed by SM EntertainmentThe group was composed of Victoria, Amber, Luna, and Krystal and previously Sulli until her departure from the group in August 15 f(x) officially debuted in September 09 with the release of the digital single "La Cha Ta" Their debut studio album, Pinocchio (11The second approach is to evaluate H(t) at ndistinct points {ti} If H(ti) = 0 for all iimplies α~= 0 then the functions {fj} are necessarily linearly independent Written in matrix form we obtain Aα~= 0 7 where Aij = fj(ti) Hence if Ais not singular then we have linear independence As in the

Behringer Neutron Self Generating Ambient Music Generative Krell Patch Pt 6 W Studio Quad 4 Fx Youtube

X Ray Hj 238 Otas F Inston Anb Utt Club Officers G S Terry President B S Burks Vice President W E Armstrong Secretary Treasurer Members W E Armstrongj M Bishopb S Burkst D Davisw

But, now suppose volume and temperature are functions of time (with n constant) V=V(t) and T=T(t) We wish to know how the pressure P is changing with time To do this we need a chain rule for functions of more than one variable We will find that the chain rule is an essential part of the solution of any related rate problemVISITORS This site recently celebrated 9 years!At t = 1, the volume V = pi r^2 h changes at a rate of A() 2 pi (b) 0 (c) e pi If xy in y = 10, then y'(x) equals (a) (y ln y)/x (b) 10(x^2 (1 ln y) (c) y/(x(1 ln y) On the circle C x(t) = 4 sin t, y(t) = 4 cos t, the rate of change of z = x^2 4y^2 8 with respect to t at r = pi/4 is (a) 48

On A Functional Equation Edinburgh Mathematical Notes Cambridge Core

Jambalaya Yearbook 1917 Ciuiii Cll Oei Ntail Club W F Rices Jr Covinglon La Melta Kappa L Ipsilnn T Tj P Inter Kralernlly Ccuiieil Tlerinau Club X M Suthon New Orleans La Ilela Thela Ii

Jan 02, 21 · \\displaystyle w=f(x,y),x=x(t,u,v),y=y(t,u,v) \nonumber\ and write out the formulas for the three partial derivatives of \(\displaystyle w\) Hint Determine the number of branches that emanate from each node in the tree AnswerIntuitively, a function is a process that associates each element of a set X, to a single element of a set Y Formally, a function f from a set X to a set Y is defined by a set G of ordered pairs (x, y) with x ∈ X, y ∈ Y, such that every element of X is the first component of exactly one ordered pair in G In other words, for every x in X, there is exactly one element y such that theMay 07, 21 · About New York Times Games Times games have captivated solvers since the launch of the Crossword in 1942 Our experts create engaging word and visual games — in 14 we introduced the Mini

Function Mathematics Wikipedia

X Ray Measurement Equipment Ptw Freiburg Gmbh

7(d) Claim f−1 f is the identity on P(A) if f is onetoone Proof Let A ∈ P(X) so A ⊆ X By 8(e) above, we know that A ⊆ f−1(f(A)) for any function f Now assume that f is onetoone Let x∈ f−1(f(A)) Then f(x) = y∈ f(A) Since y ∈ f(A), there exists a ∈ A such that f(a) = y Since f(x) = y = f(a) and f is onetooneAnswer Clear Kahn Academy is a free site with videos of a guy explaining this stuff clearly Here is their video explaining W=Fd If you're still confused, look at their other videos on the subject hereP ∈ R3 is the ordered triple (r,θ,z) defined by the picture y z x 0 P r z Remark Cylindrical coordinates are just polar coordinates on the plane z = 0 together with the vertical coordinate z Theorem (Cartesiancylindrical transformations) The Cartesian coordinates of a point P = (r,θ,z) are given by x = r cos(θ), y = r sin(θ), and z = z

A Work Energy And Power

Docx Tsinghua Math Camp 15

P(x)dx and p X(x) = p(x) = F0(x) The following are all equivalent X˘P;Looking for online definition of F/P/T or what F/P/T stands for?JP Morgan Strategic Volatility Dynamic Index1,8 0 0 OVERVIEW The JP Morgan Strategic Volatility Dynamic Index (the "Index") aims to provide exposure to volatility by combining a long position and a contingent short position in futures contracts on the CBOE Volatility Index(R) (the "VIX") futures curve

D B 0 B

Publications Tsung Group

This list of all twoletter combinations includes 1352 (2 × 26 2) of the possible 2704 (52 2) combinations of upper and lower case from the modern core Latin alphabetA twoletter combination in bold means that the link links straight to a Wikipedia article (not a disambiguation page) As specified at WikipediaDisambiguation#Combining_terms_on_disambiguation_pages,You are the following visitor number, since 5/29/97This problem has been solved!

Mo Pt Overlayers As Efficient Catalysts For Hydrodeoxygenation Of Guaiacol And Anisole Catalysis Science Technology Rsc Publishing

Pdf An Approximation Of Partial Sums Of Independent Rv S And The Sample Df I

MATH 110 LINEAR ALGEBRA HOMEWORK #3 FARMER SCHLUTZENBERG §21 Linear Transformations, Null Spaces, and Ranges Problem 1 Here V and W are vector spaces over a field F and T V → W (but T may not be linear) (a) TrueP = mv kgm/s Impulse Momentum Dp = FDt kgm/s Momentum Conservation p 1 p 2 = p' 1 p' 2 kgm/s Work W = Fd J or Nm Power P = W/t J/s or W Power P = Fv J/s or W Power P = Τω J/s or W Kinetic Energy KE = (1/2)mv 2 J Potential Energy PE = mgh J Pressure p = F/A Pa Pressure (fluid) p = rhg Pa Pascal's PrincipalAnd, if you don't have cable, here are some (mostly) free ways to stream MLB games Watch MLB Baseball's Free Live Streams for Fans Without Cable Uncategorized RuPaul's Drag Race is a cultural icon, but it wasn't the first show to break gender identity and crossdressing barriers Robin Williams, Ving Rhames, Hilary Swank and many others

Perturbation Analysis For The Matrix Scaled Total Least Squares Problem

Solved Question 3 Determine Formula For Wut Of The Follo Chegg Com

Mar 10, 21 · to be any number we get the following formula for computing directional derivatives D→uf(x, y) = fx(x, y)a fy(x, y)b D → u f ( x, y) = f x ( x, y) a f y ( x, y) b This is much simpler than the limit definition Also note that this definition assumed2 If w = f(x 1,x 2,···,x m) and for each i, (1 ≤ i ≤ n), x i = x i(t 1,t 2,···,t n) such that f,x 1,···,x m are all differentiable Then ∂w ∂t i = ∂w ∂x 1 ∂x 1 ∂t i ··· ∂w ∂x m ∂x m ∂t i (2) Example (1) Given w = ln(u v z), with u = cos2 t, v = sin2 t and z = t2, findT t Figure 36 z= f(x(t);y(t)) Similarly, if w= f(x;y;z) and x;y;zare functions of t, then the corresponding tree structure is shown in –gure 37 Again, wis ultimately a function of t So, there is only one derivative to compute, dw dt Using the interpretation outlines above, we obtain the following formula dw dt = @w @x dx dt @w @y dy

Wonderland Model Wikipedia

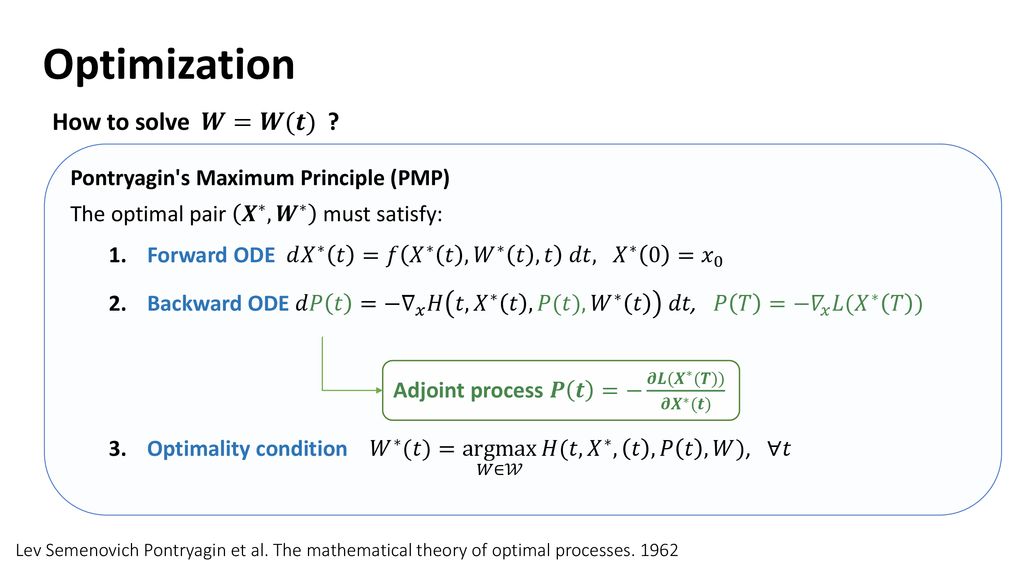
Ordinary Differential Equations And Machine Learning Ppt Download

2 Pc Combo Deal Inferno Pt 4 2 0 Krueger Silicone Freddy Mask Etsy

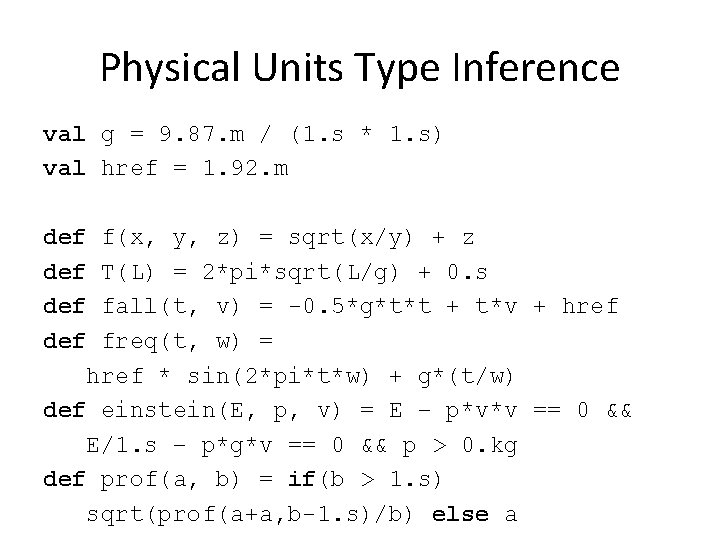
Type Inference Def Constx T Lst Listt Listt

Derivative Calculator Wolfram Alpha

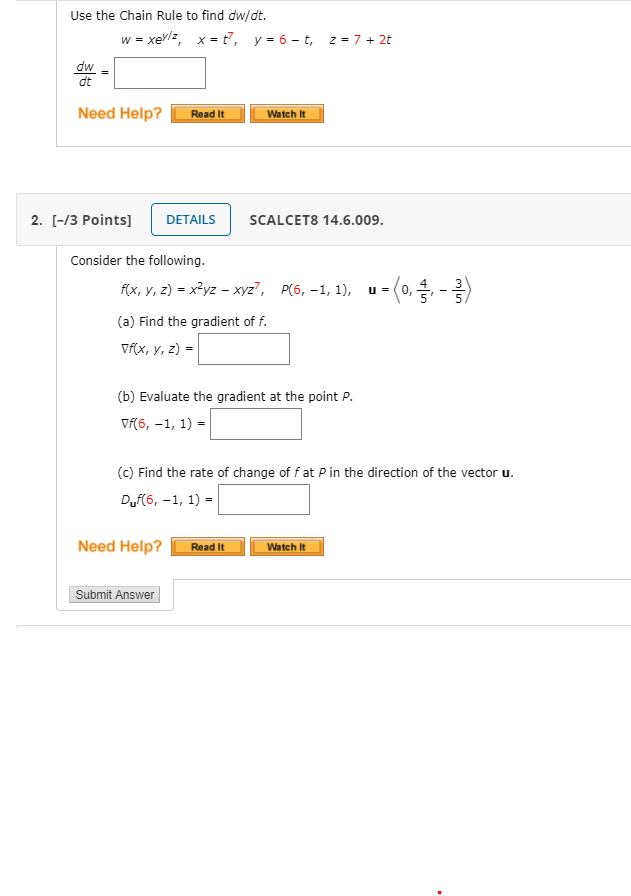
Solved 1 Use The Chain Rule To Find Dw Dt W Xey Z X Chegg Com

Let P W Be The Fourier Transform Of P T Let Q Be The Fourier Transform Of Q T We Want To Be Sure In Our Models And Analysis Not To Make

Gradient Wikipedia

Calc 3 Cheat Sheet Mathematical Analysis Space

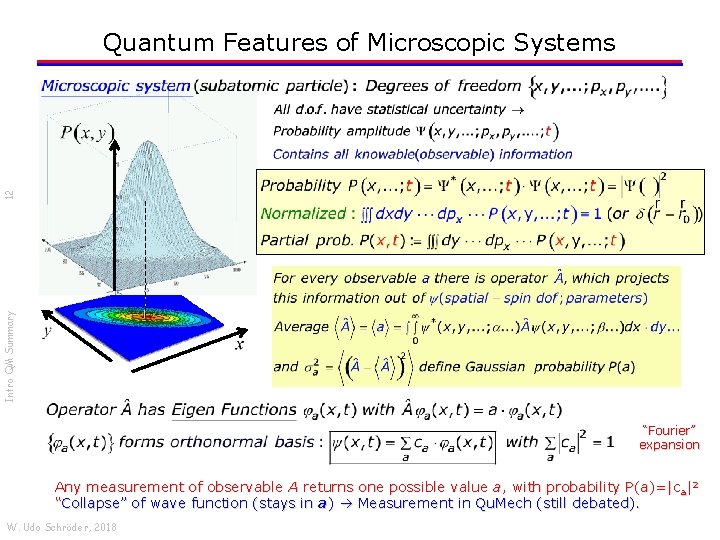
The Quantum World Intro Qm Summary 2 Particlewave

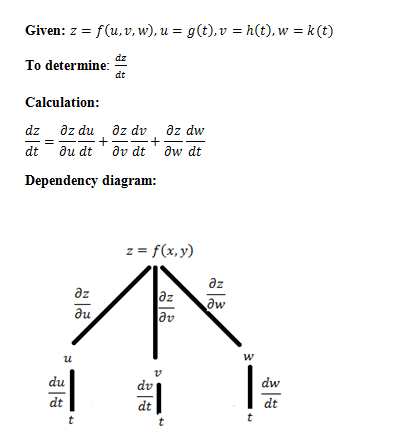
Answered For Z 13 Dt F X Y X G T Y Bartleby

Fx Coudert Nice And If You Like Naturechemistry S In Your Element Essays Here Is An Up To Date Periodic Table With All Of Them Linked T Co Nu4yzx0wkb T Co O7eskstu5s

Derivative Of Composite Function And Chain Rule Mathematics Stack Exchange

Specialized Welding Solutions Kemppi

Use Adjoint Process To Compute Gradient With Low Memory Cost Download Scientific Diagram

How To Teach Blending Sounds To Read Words Reading Simplified

Pdf Recursive Bayesian Inference On Stochastic Differential Equations

Work Calculator Work Formula Work Units

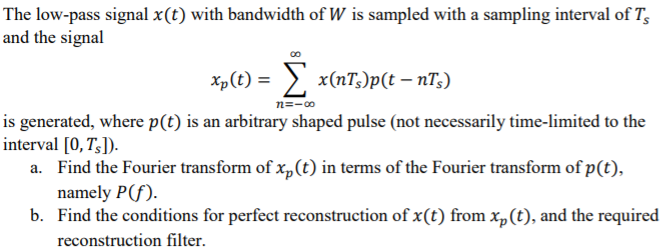
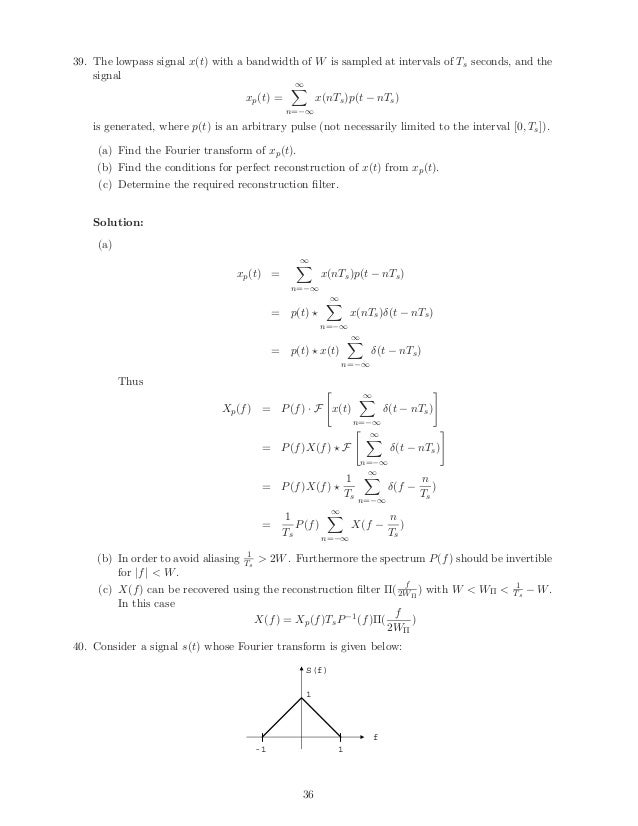
Solved The Low Pass Signal X T With Bandwidth Of W Is Sa Chegg Com

X Ray Nia Nelson William Virginia Otey W M Virginia Parker P G North Carolina Parker W R North Carolina Parson A D Virginia Peery V P Virginia Phillips Charles

Solved If W F X Y Z Where X G S T Y H S T Chegg Com

Compute Partial Derivatives With Chain Rule

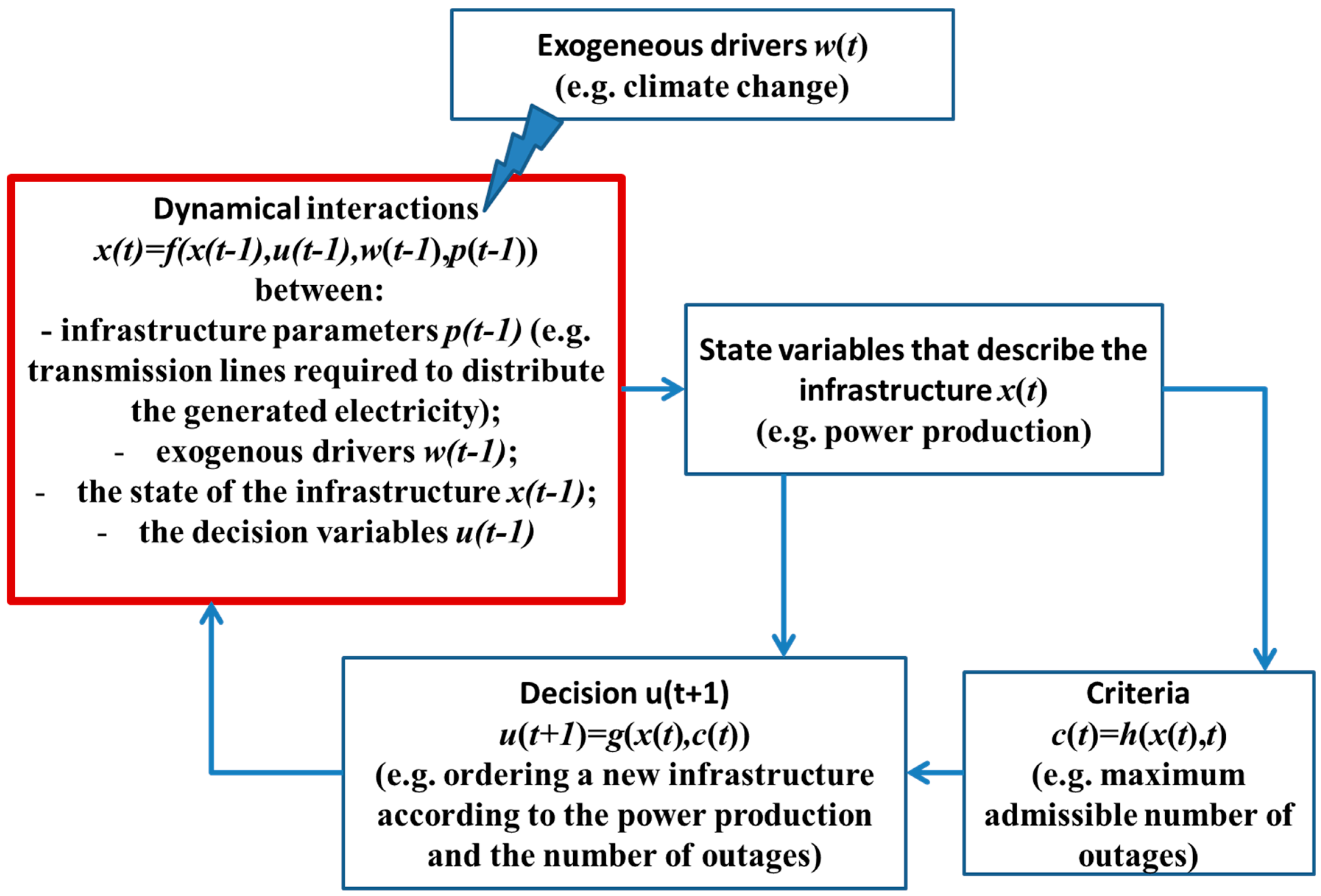
Infrastructures Free Full Text An Integrated Dynamical Modeling Perspective For Infrastructure Resilience Html

Use The Chain Rule To Find The Indicated Partial Derivatives P U2 V2 W2 U Xey V Yex W Exy P X P Y

Solution Matematikcentrum

Specialized Welding Solutions Kemppi

Pin On Foreign Languages

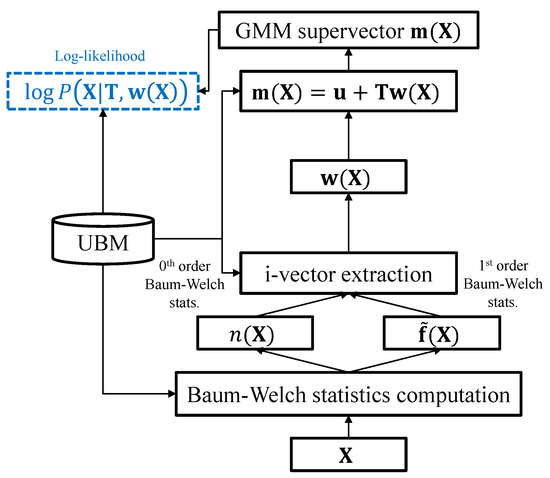
Sensors Free Full Text Adversarially Learned Total Variability Embedding For Speaker Recognition With Random Digit Strings Html

Solvedproblems Phpapp01

Amazon Com Pedaltrain Pro Fx Pedalboard With Soft Case 5 Rails 32 X 16 Pt Pdb Pfx Sc Musical Instruments

Csci 52 Natural Language Processing Jim Martin Lecture

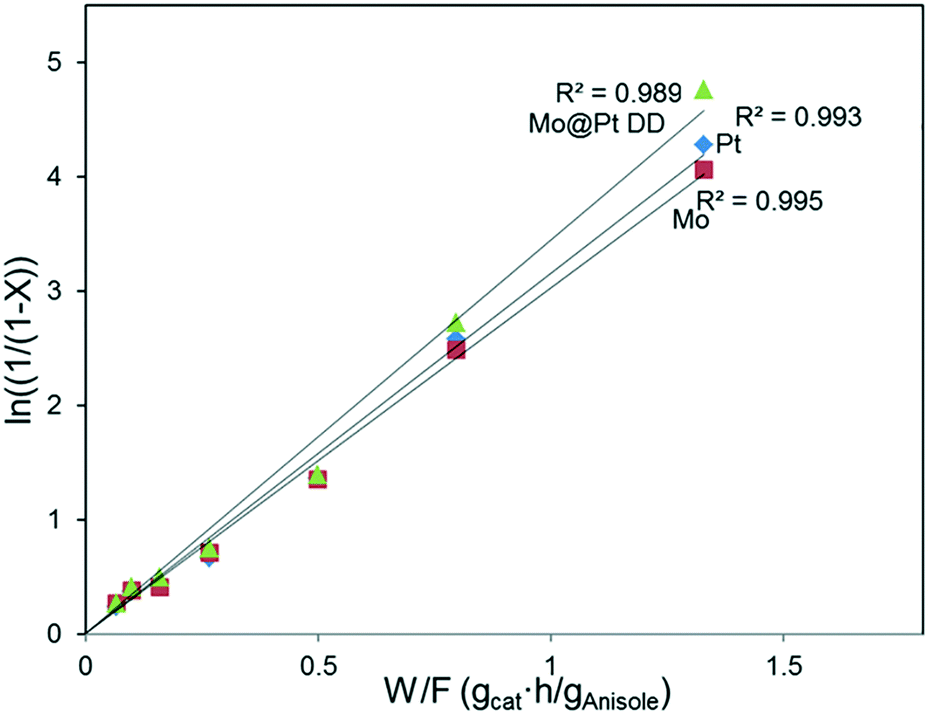
Conversion Of A M X T 623 K P 0 101 Mpa W F 0 25 47 Kg S Mol Download Scientific Diagram

Soft X Ray Chambers Ptw Freiburg Gmbh

Conversion Of A M X T 623 K P 0 101 Mpa W F 0 25 47 Kg S Mol Download Scientific Diagram

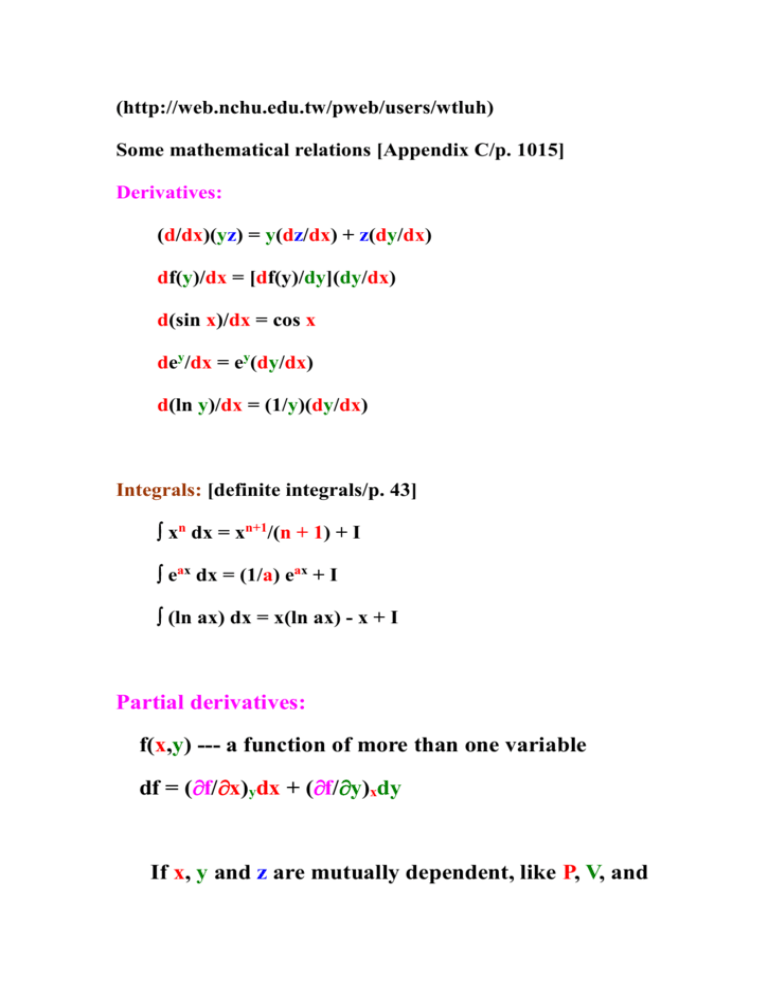
Some Mathematical Relations Appendix C P

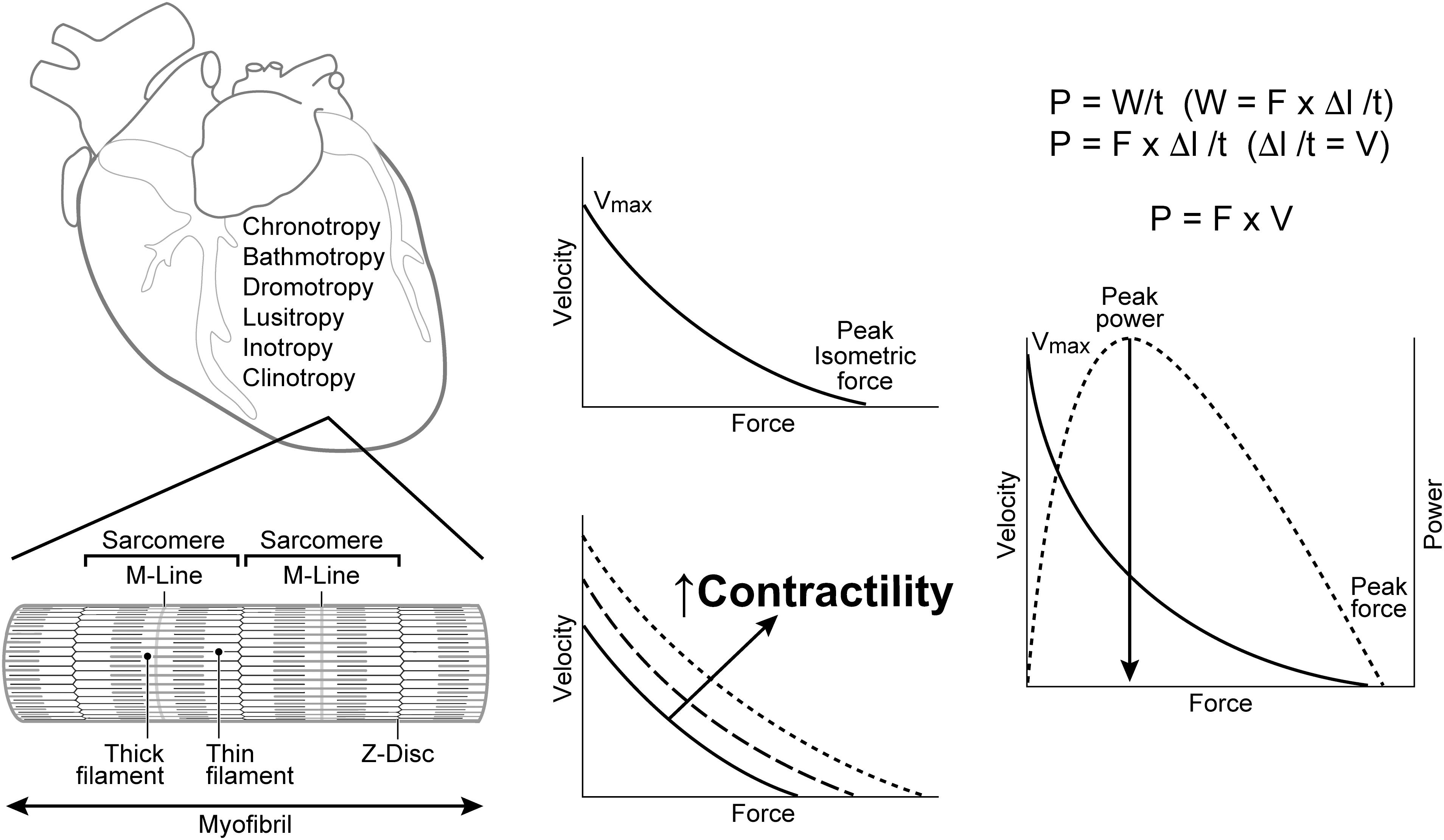
Frontiers Myocardial Contractility Historical And Contemporary Considerations Physiology

Work Physics Wikipedia

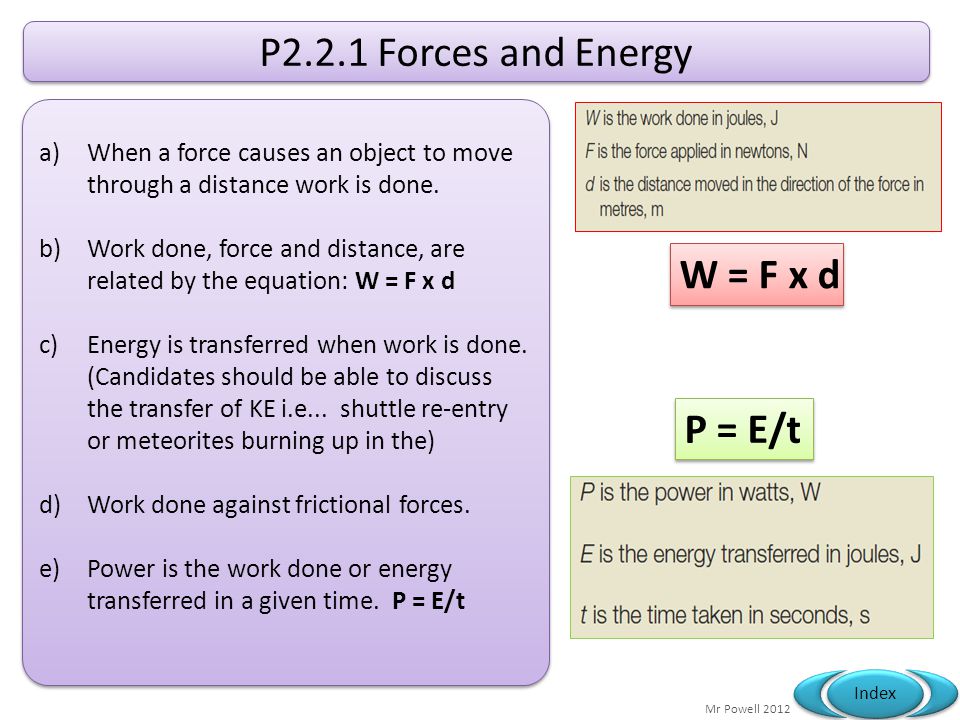
P2 2 1 Forces And Energy P2 Physics Ks4 Additional Science Mr D Powell Ppt Download

Solved 6 Consider The Following Second Order Linear Init Chegg Com

Equation Sheet Final Test Phys 1171 Lab For Physics 1171 Studocu

Worksheet Optimization Printable Worksheets Activities Ulus Answers Chter Review Ans Pt Fun Multiplication Games Kids Grade Work Mathematical Ab Place Free Calculus Sumnermuseumdc Org

Mize Flowstate Giveaway Time Ef X Wf X Mize Rules Rt Like Follow Mizedub Wobble Factory Electric Hawk Reply To This Tweet With A There Will Be A

1 Vytah

Calyx W F Slater Ata H X Smith W C Smith J A Spaxx D K Staxton Jr L T Starbird D L Sterxberger Z B Tay M Stevexs S A

Engineering Design By Geometric Programming Topic Of Research Paper In Mathematics Download Scholarly Article Pdf And Read For Free On Cyberleninka Open Science Hub

Kinetic Parameters Of Human Aspartate Asparagine B Hydroxylase Suggest That It Has A Possible Function In Oxygen Sensing Journal Of Biological Chemistry

No You Are Not Getting Paid For This Ppt Download